Van T Hoff Factor Table
13.9: Solutions of Electrolytes
- Page ID
- 24261
Thus far nosotros take causeless that nosotros could just multiply the molar concentration of a solute past the number of ions per formula unit to obtain the actual concentration of dissolved particles in an electrolyte solution. Nosotros have used this simple model to predict such backdrop as freezing points, melting points, vapor force per unit area, and osmotic pressure. If this model were perfectly right, we would await the freezing point depression of a 0.10 1000 solution of sodium chloride, with 2 mol of ions per mole of \(NaCl\) in solution, to be exactly twice that of a 0.10 m solution of glucose, with only 1 mol of molecules per mole of glucose in solution. In reality, this is not always the case. Instead, the observed change in freezing points for 0.10 m aqueous solutions of \(NaCl\) and KCl are significantly less than expected (−0.348°C and −0.344°C, respectively, rather than −0.372°C), which suggests that fewer particles than we expected are present in solution.
The relationship between the actual number of moles of solute added to form a solution and the apparent number as determined by colligative properties is called the van't Hoff factor (\(i\)) and is defined every bit follows:Named for Jacobus Hendricus van't Hoff (1852–1911), a Dutch chemistry professor at the University of Amsterdam who won the showtime Nobel Prize in Chemistry (1901) for his work on thermodynamics and solutions.
\[i=\dfrac{\text{credible number of particles in solution}}{\text{ number of moles of solute dissolved}} \label{13.nine.one}\]
As the solute concentration increases the van't Hoff cistron decreases.
The van't Hoff factor is therefore a measure out of a deviation from ideal beliefs. The lower the van 't Hoff factor, the greater the deviation. As the data in Table \(\PageIndex{i}\) testify, the van't Hoff factors for ionic compounds are somewhat lower than expected; that is, their solutions apparently contain fewer particles than predicted past the number of ions per formula unit. As the concentration of the solute increases, the van't Hoff factor decreases because ionic compounds more often than not do not totally dissociate in aqueous solution.
| Compound | i (measured) | i (platonic) |
|---|---|---|
| glucose | ane.0 | one.0 |
| sucrose | i.0 | ane.0 |
| \(NaCl\) | 1.9 | two.0 |
| \(HCl\) | 1.nine | ii.0 |
| \(MgCl_2\) | 2.7 | 3.0 |
| \(FeCl_3\) | 3.4 | iv.0 |
| \(Ca(NO_3)_2\) | two.5 | 3.0 |
| \(AlCl_3\) | 3.ii | 4.0 |
| \(MgSO_4\) | 1.4 | 2.0 |
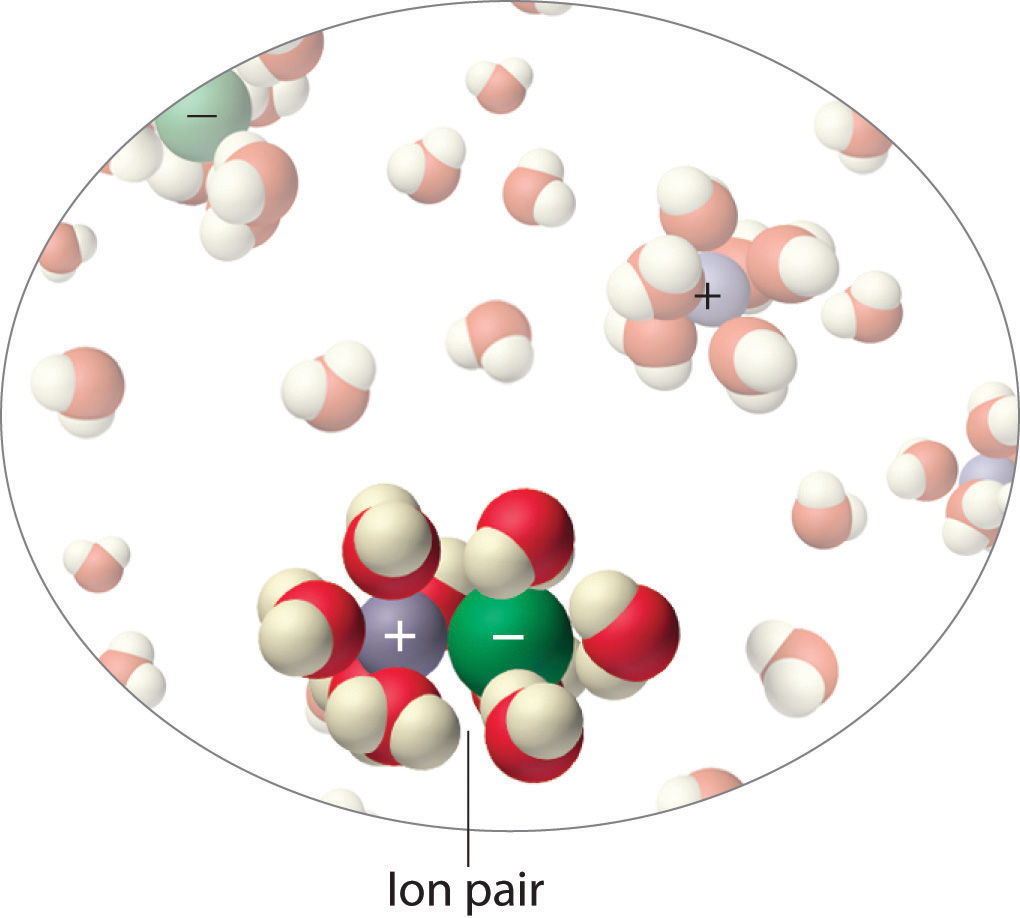
Instead, some of the ions exist equally ion pairs, a cation and an anion that for a cursory time are associated with each other without an intervening trounce of h2o molecules (Effigy \(\PageIndex{1}\)). Each of these temporary units behaves similar a single dissolved particle until it dissociates. Highly charged ions such as \(Mg^{2+}\), \(Al^{three+}\), \(\ce{SO4^{ii−}}\), and \(\ce{PO4^{3−}}\) take a greater tendency to form ion pairs because of their strong electrostatic interactions. The actual number of solvated ions present in a solution can be determined by measuring a colligative belongings at several solute concentrations.
Example \(\PageIndex{1}\): Iron Chloride in Water
A 0.0500 One thousand aqueous solution of \(FeCl_3\) has an osmotic pressure level of 4.15 atm at 25°C. Summate the van't Hoff factor \(i\) for the solution.
Given: solute concentration, osmotic pressure, and temperature
Asked for: van't Hoff gene
Strategy:
- Utilize Equation 13.9.12 to calculate the expected osmotic pressure of the solution based on the effective concentration of dissolved particles in the solvent.
- Calculate the ratio of the observed osmotic pressure to the expected value. Multiply this number by the number of ions of solute per formula unit of measurement, and then use Equation 13.nine.ane to calculate the van't Hoff cistron.
Solution:
A If \(FeCl_3\) dissociated completely in aqueous solution, it would produce four ions per formula unit of measurement [Fe3+(aq) plus 3Cl−(aq)] for an effective concentration of dissolved particles of 4 × 0.0500 Chiliad = 0.200 M. The osmotic pressure would exist
\[\Pi=MRT=(0.200 \;mol/50) \left[0.0821\;(L⋅atm)/(One thousand⋅mol) \right] (298\; K)=4.89\; atm\]
B The observed osmotic pressure is but 4.15 atm, presumably due to ion pair formation. The ratio of the observed osmotic pressure to the calculated value is 4.fifteen atm/iv.89 atm = 0.849, which indicates that the solution contains (0.849)(four) = 3.40 particles per mole of \(FeCl_3\) dissolved. Alternatively, we tin summate the observed particle concentration from the osmotic pressure of four.15 atm:
\[4.15\; atm=K \left[ 0.0821 \;(50⋅atm)/(K⋅mol)\right] (298 \;One thousand) \]
\[0.170 mol/L=Thou\]
The ratio of this value to the expected value of 0.200 M is 0.170 M/0.200 Grand = 0.850, which again gives united states of america (0.850)(4) = 3.40 particles per mole of \(FeCl_3\) dissolved. From Equation \ref{thirteen.9.1}, the van't Hoff factor for the solution is
\[i=\dfrac{\text{iii.40 particles observed}}{\text{1 formula unit}\; FeCl_3}=three.40\]
Exercise \(\PageIndex{one}\): Magnesium Chloride in Water
Calculate the van't Hoff factor for a 0.050 m aqueous solution of \(MgCl_2\) that has a measured freezing point of −0.25°C.
Answer:
2.7 (versus an platonic value of 3
Key Concepts and Summary 
Ionic compounds may non completely dissociate in solution due to activity effects, in which case observed colligative furnishings may be less than predicted.
Van T Hoff Factor Table,
Source: https://chem.libretexts.org/Bookshelves/General_Chemistry/Map%3A_General_Chemistry_(Petrucci_et_al.)/13%3A_Solutions_and_their_Physical_Properties/13.09%3A_Solutions_of_Electrolytes
Posted by: padulaablumersy.blogspot.com


0 Response to "Van T Hoff Factor Table"
Post a Comment